Spin angular momentum

State functions are eigenvalues of the Sz and S2 operators. The derivation of the expectation value of the S2 operator is given in this section.

The fundamental spin operators have the following effects:

Sxα = ½β

Sxβ = ½α
Syα = i½β Syβ = -i½α
Szα = ½α Szβ = -½β
 

Using these expressions, various useful identities can be established:

S2 = Sx2 + Sy2 + Sz2
I+ = (Sx + iSy) I+β = α I+α = 0
I- = (Sx - iSy) I-α = β I-β = 0

 



Sx2+Sy2 = (I+I-)+i(SxSy-SySx)
  = (I-I+)+i(SySx-SxSy)
  = ½(I+I- + I-I+)

and finally i(SySx - Sx Sy ) = Sz.

For any microstate Ψ, the expectation value of the S2 operator is given by    <S2> = <Ψ|Sz2 + Sy2 + Sx2|Ψ>

 
The first part of this expression is obvious, vis:    <Ψ|Sz2|Ψ> = ¼(Nα + Nβ)
 
However, the effect of Sy2+Sx2 is not so simple. By making use of the fact that the operators involve two electrons, a large number of integrals resulting from the expansion of the Slater determinants can be readily eliminated. The only integrals which are not zero due to the orthogonality of the eigenvectors, i.e., those which may be finite due to the spin operators, are
\begin{displaymath}<\Psi\vert S_y^2+S_x^2\vert\Psi> = 2\sum_{i<j}[<\psi_i\psi_i\... ...i_j\psi_j>- <\psi_i\psi_j\vert S_y^2+S_x^2\vert\psi_i\psi_j>]. \end{displaymath}

Using the relationships already defined, this expression simplifies [65] as follows:
\begin{displaymath}S_1S_2=S_{1z}S_{2z}+\frac{1}{2}(I_1^+I_2^-+I_1^-I_2^+) \end{displaymath}

\begin{displaymath}<\Psi\vert S^2\vert\Psi> = 2\sum_{i<j}[\frac{1}{4}(2\delta(m_... ...s_j} -1-\frac{1}{2}(1-\delta(m_{s_i}m_{s_j}))<\psi_i\psi_j>^2] \end{displaymath}

or,
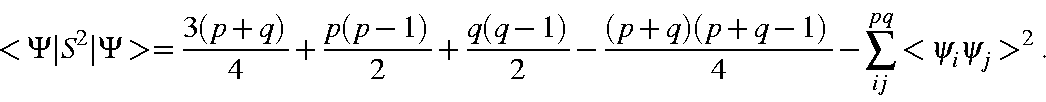
\begin{displaymath}<\Psi\vert S^2\vert\Psi> =\frac{3(p+q)}{4}+\frac{p(p-1)}{2}+\... ...1)}{2} -\frac{(p+q)(p+q-1)}{4}-\sum_{ij}^{pq}<\psi_i\psi_j>^2. \end{displaymath}

Recall that p is the number of α electrons, and q, the number of β electrons. This expression simplifies to yield
\begin{displaymath}<\Psi\vert S^2\vert\Psi> =\frac{1}{2}(p+q)+\frac{1}{4}(p-q)^2- \sum_i^p\sum_j^q<\psi_i\psi_j>^2. \end{displaymath}

For the general case, in which the state function Φ, is a linear combination of microstates, the expectation value of S is more complicated:
\begin{displaymath}<\Phi_k\vert S^2\vert\Phi_k> = \sum_i\sum_jC_{ik}C_{jk}<\Psi_i\vert S^2\vert\Psi_j> . \end{displaymath}
As with the construction of the C.I. matrix, the elements of this expression can be divided into a small number of different types:
1.  Ψa=Ψb: Since the two wavefunctions are the same, this corresponds to the expectation value of a microstate, and has already been derived.
2.  Except for ψi  in Ψa and ψj in Ψb; Ψa=Ψb: Assuming ψi and ψj  to have alpha-spin the expectation value is
\begin{displaymath}<\Psi_a\vert S_y^2+S_x^2\vert\Psi_b>= (-1)^W\sum_{k=B}^A(<ij\vert kk>-<ik\vert jk>)O_k^{\alpha a} +<ij\vert kk>O_k^{\beta a}. \end{displaymath}

The effect of the spin operator is to change the spin of the electrons but leave the space part unchanged. All integrals vanish identically due to one or more of the following identities:

 

<ψiψj>  =  <mimi>  =  Δ(i,j)
<ψiψk>  = Δ(i,k)    
<ψiψk>  =  Δ(j,k)    
Therefore, <Ψa|S2|Ψb> = 0.
3. Except for ψi and ψj in Ψa and ψk and ψl in Ψb; Ψa=Ψb. Two situations exist: (a) when all four M.O.s are of the same spin; and (b) when two are of each spin.

When all four M.O.s have the same spin, the effect of the spin operator is to reverse the spin of two M.O.s in the ket half of the integral. By spin orthogonality this results in an integral value of zero.

In the case where two M.O.s are of α spin and two are of β spin, the matrix elements, after elimination of those terms which are zero due to space orthogonality, are

\begin{displaymath}<\Psi_a\vert S^2\vert\Psi_b> = (-1)^W(<\psi_i\psi_k\vert S^2\vert\psi_j\psi_l>- <\psi_i\psi_l\vert S^2\vert\psi_j\psi_k>) \end{displaymath}
The effect of S2 on ψk and ψl is to reverse the spin of these functions; this gives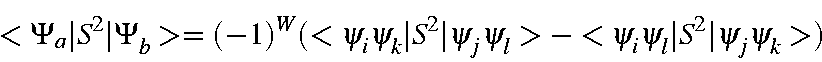
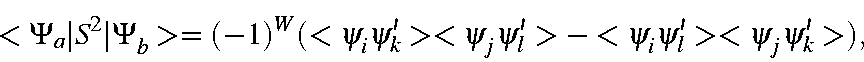
\begin{displaymath}<\Psi_a\vert S^2\vert\Psi_b> = (-1)^W(<\psi_i\psi_k'><\psi_j\psi_l'>- <\psi_i\psi_l'><\psi_j\psi_k'>) , \end{displaymath}

where ψ' has the opposite spin to that of ψ.

Thus, only if  ψi and ψj are spatially identical with ψk and ψl  will <Ψa|S2|Ψb> be non-zero. The phase-factor W is such that if i=k and j=l then W=-1, and if i=l and j=k then W=1; for all other cases the matrix element is zero, so the phase of W is irrelevant. For these two cases, the matrix element is <Ψa|S2|Ψb> = 1 if $(I^++I^-)(\psi_i+\psi_j)=(\psi_k+\psi_l)$, otherwise <Ψa|S2|Ψb> = 0.

4. If more than two differences exist, <Ψa|S2|Ψb> = 0.