NDDO two-electron two-center integrals

In a local diatomic frame there are 22 unique two-electron two-center integrals for each pair of heavy (non-hydrogen) atoms. These are shown in Table 1.


 

Table 1: Two-Electron Two-Center Integrals (Local Frame)

1

<ss|ss>

12

<s  ps|pp  pp>

2

<ss|pp  pp>

13

<s  ps|ps ps> 

3

 <ss|ps ps>

14

<ss|s ps>

4

 <pp  pp|ss>

15

<pp  pp|s  ps> 

5

<ps ps|ss>

16

<ps  ps|s  ps>

6

<pp  pp|pp  pp>

17

<s  pp|s  pp>

7

<pp  pp|pp'  pp'  >

18

<s  ps|s  ps> 

8

<pp  pp|ps  ps>

19

<s  pp| pp ps> 

9

<ps  ps|pp  pp>

20

<pp ps| s  pp>  

10

<ps  ps|ps  ps> 

21

<pp ps|  pp ps>   

11

<s ps|ss>

22

<pp  pp' |pp pp'  >


Each integral represents the energy of an electron density distribution (electron 1) arising from the product of the first two atomic orbitals interacting with the electron density distribution (electron 2), which in turn arises from the product of the second two atomic orbitals. Only if the first two atomic orbitals are the same and the second two are the same will the interaction energy have to be positive, in which case the integral represents an electron-electron repulsion term. In all other cases the sign of the integral value may be positive or negative.

With the exception of integral 22, all the integrals can be calculated using different techniques without loss of rotational invariance. That is, no integral depends on the value of another integral, except for number 22. As with the Hpp monocentric integral, it is easy to show that:

<pp  pp' |pp pp'  > = 1/2(<pp  pp|pp  pp>  - <pp  pp|pp'  pp'  >).

The electron density distributions are approximated by a series of point charges. There are four possible types of distribution. These are given in Table 2.

    

Table 2: Types of Electron Density Distribution

Monopole

Unit negative charge centered on the

(1 charge)

nucleus

 

 

Dipole

+1/2 charge located at position (x,y,z),

(2 charges)

-1/2 charge located at position (-x,-y,-z)

 

 

Linear Quadrupole

+1/2 charge located at the nucleus, -1/4 charge

(3 charges)

at positions (x,y,z) and at (-x,-y,-z)

 

 

Square Quadrupole

Four charges of magnitude +1/4, -1/4, +1/4

(4 charges)

and -1/4 forming a square centered on the nucleus.

These are used to represent the four types of atomic orbital products (Table 3).


 

Table 3: Density Distributions Arising from Pairs of Atomic Orbitals

Atomic Orbitals

Multipole Distribution

Number of Charges

<ss|

Monopole

1

<sp|

Dipole

2

<pp|

Monopole plus Linear Quadrupole

4

<pp'|

Square Quadrupole

4


Each two electron interaction integral is the sum of all the interactions arising from the charge distribution representing one pair of atomic orbitals with the charge distribution representing the second pair of atomic orbitals. Thus, in the simplest case, the <ss|ss> interaction is represented by the repulsion of two monopoles, while a <pp pp |pp ' pp'> , a much more complicated interaction, is represented by 16 separate terms, arising from the four charges representing the monopole and linear quadrupole on one center interacting with the equivalent set on the second center.

While the repulsion of two like charges is proportional to the inverse distance separating the charges, boundary conditions preclude using this simple expression to represent the interelectronic interactions. Instead, the interaction energy is approximated by:

All that remains is to specify functional forms for the terms c and A. c, the distance of a multipole charge from its nucleus, is a simple function of the atomic orbitals; in the case of a s-p product, this is a vector of length D1 Bohr pointing along the p axis, where

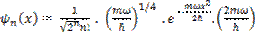
begin{displaymath}D_1 = frac{(2n+1)(4xi_sxi_p)^{(n+1/2)}}{sqrt{3}(xi_s+xi_p)^{(2n+2)}}.end{displaymath}

The principal quantum number is always the same in these methods for s and p orbitals on any given atom. The corresponding distances of the charges from the nucleus for the linear and square quadrupoles are 2D2 and 21/2D2 Bohr, respectively, where

begin{displaymath}D_2=left(frac{4n^2+6n+2}{20}right)^{1/2}frac{1}{xi_p}.end{displaymath}

Now that the distances of the charges from the nucleus have been defined, the upper boundary condition can be set. In the limit, when R=0.0, the value of the two-electron integral should equal that of the corresponding monocentric integral. Three cases can be identified:

1. A monopole-monopole interaction, in which case the integral must converge on Gss.
2. A dipole-dipole interaction, where the integral must converge on Hsp.
3. The quadrupole-quadrupole interaction where the integral must converge on Hpp.

For convenience, the GA terms are given special names. These are given in Table 4.    

Table 4: Additive Terms for Two-Electron Integrals

Multipole

Monocentric Equivalent

Name

Monopole

Gss

AM

Dipole

Hsp

AD

Quadrupole

Hpp =1/2(Gpp-Gp2)

AQ


In practice, 1/2(Gpp - Gp2) is used instead of Hpp. This eliminates any possibility of loss of rotational invariance due to an incorrect value of Hpp.

While AM is given simply by Gss/27.21, AD and AQ are complicated functions of one-center terms and the orbital exponents--recall that, in the limit, the associated charges are not all coincident. AD and AQ are solved iteratively. Given an initial estimate of AD of

begin{displaymath}AD = left[frac{H_{sp}}{27.21D^2_1}right]^{1/3},end{displaymath}

then, by iterating, an exact value of AD can be found. On iteration n the value of AD is given by

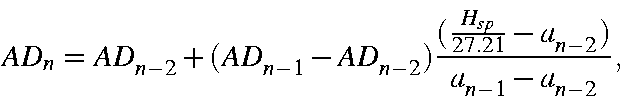
begin{displaymath}AD_n = AD_{n-2}+(AD_{n-1}-AD_{n-2})frac{(frac{H_{sp}}{27.21}-a_{n-2})}{a_{n-1}-a_{n-2}},end{displaymath}

where

an=1/2ADn-1/2(4D21+AD-2n)-1/2.

About 5 iterations are needed in order to get AD specified with acceptable accuracy.

Similarly, for AQ an initial estimate of $[frac{H_{pp}}{27.21(3D^4_2)}]^{1/5}$is made and, again, by iterating using

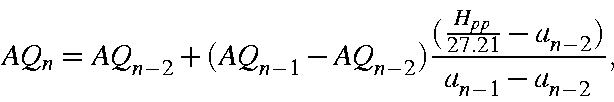
begin{displaymath}AQ_n = AQ_{n-2}+(AQ_{n-1}-AQ_{n-2})frac{(frac{H_{pp}}{27.21}-a_{n-2})}{a_{n-1}-a_{n-2}},end{displaymath}

where, now,

an=1/4AQn - 1/2(4D22+AQ-2n)-1/2 + 1/4(8D22+AQn-2)-1/2,

an exact value of AQ can be obtained. About 5 iterations are necessary.

Note that these equations are intrinsically unstable on finite-precision computers. The denominator (an-1 -an-2 ) rapidly becomes vanishingly small; this is, however, necessary in order to accurately define AD and AQ.